PROBN

Purpose:

Returns the probability of X <= z for a normal distribution.

Syntax:

PROBN(z, mean, std)

z	-	A real or series. The z value.
mean	-	Optional. A real, the mean of the distribution. Defaults to 0.0.
std	-	Optional. A real, the standard deviation of the distribution, where std > 0. Defaults to 1.0.

Returns:

A real or series, the probability that a value is less than or equal to the input z value for a normal distribution with the given mean and standard deviation.

For the input value z, returns the probability p where P(X <= z) = p.

Example:

probn(0)

returns 0.5, the probability that a value is less than or equal to 0.0 for a normal distribution with a mean of 0.0 and a standard deviation of 1.0.

In probabilistic terms, given the normal distribution N(0, 1), i.e. mean of 0, variance of 1:

P(X <= 0.0) = 0.5

Example:

probn(2, 1, 2) - probn(0, 1, 2)

returns 0.382925, the probability that a value is less than or equal to 2 and greater than or equal to 0.0 for a normal distribution with a mean of 1.0 and a standard deviation of 2.0

In probabilistic terms, given the normal distribution N(1, 4), i.e. mean of 1, variance of 4:

P(0.0 <= X <= 2.0) = 0.382925

Example:

1 – probn(.5)

returns 0.30853754, the probability that a value is greater than 0.5 for a normal distribution with a mean of 0.0 and a standard deviation of 1.0

Example:

probn(invprobn(.3))

returns 0.3, indicating that PROBN and INVPROBN are inverse functions.

Example:

probn(-3..0.01..3)

displays the normal cumulative distribution function from –3 to 3.

Remarks:

The result is NaN where std ≤ 0.

The probability density function, f(x), for normally distributed random values is:

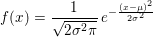
where μ is the mean, σ is the standard deviation and σ² is the variance. The cumulative distribution function, Φ(x), is:

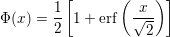
where erf(x) is the error function implemented by ERF. PROBN uses ERF to evaluate the area under the normal distribution curve. Note that probn(z) returns the area from -∞ to z.

Approximately 68% of the values from a normal distribution are within one standard deviation away from the mean. Approximately 95% of the values lie within two standard deviations and approximately 99.7% are within three standard deviations. This is known as the 3-sigma rule.

See INVPROBN to calculate the inverse normal cumulative distribution function. PROBN and INVPROBN are inverse functions.

See PDFNORM to generate the normal density function.

See GNORMAL to generate a series of normally distributed random values.

PROBN

Purpose:

Syntax:

Returns:

Example:

Example:

Example:

Example:

Example:

Remarks:

See Also: